|
This part of the lesson details signs of comparison compounded vertically and horizontally.
Signs of Comparison Compounded Vertically: When two or more simple signs of comparison are arranged one under the other, the combination becomes a single comparison sign compounded vertically. The symbol for the uppermost sign must be written first and unspaced from the symbol for the next lower sign.
- Arrows:
When a right pointing arrow in regular type with a full barb and single shaft of ordinary length is part of a sign of comparison compounded vertically, its contracted form must not be used.
| Pointing-Right Over Pointing-Left |
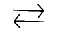 |
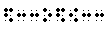 |
| Pointing-Right Over Pointing-Left |
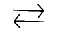 |
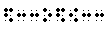 |
| Pointing-Left Over Pointing-Right |
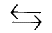 |
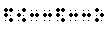 |
Long Pointing-Right
Over Short Pointing-Left |
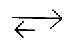 |
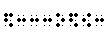 |
Short Pointing-Right
Over Long Pointing-Left |
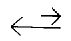 |
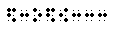 |
Pointing-Right With Upper Barb Only
Over Pointing-Left With Lower Barb Only |
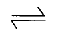 |
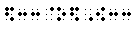 |
Pointing-Left Over
Boldface Pointing-Right |
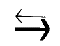 |
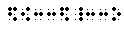 |
Boldface Pointing-Right
Over Pointing-Left |
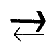 |
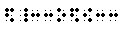 |
Boldface Pointing-Left
Over Pointing-Right |
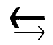 |
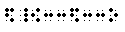 |
Boldface Pointing-Right
Over Boldface Pointing-Left |
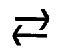 |
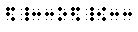 |
Boldface Pointing-Left
Over Boldface Pointing-Right |
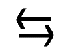 |
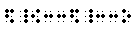 |
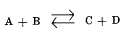
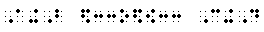
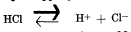
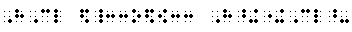
- Equals:
| Horizontal Bar |
- |
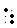 |
| Oblique Stroke |
/or\ |
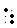 |
| Equals Sign |
= |
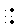 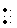 |
As shown in c through k below, a single horizonal bar, an oblique stroke, or an equals sign may appear as part of a sign of comparison compounded vertically. The horizontal bar or oblique stroke is often substituted for the equals sign.
- Greater Than:
Bar Over Greater Than
(is equal to or greater than) |
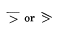 |
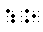 |
Equals Sign Over Greater Than
(is equal to or greater than) |
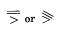 |
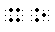 |
Bar Under Greater Than
(is greater than or equal to) |
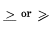 |
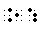 |
Equals Sign Under Greater Than
(is greater than or equal to) |
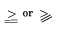 |
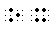 |
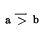
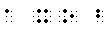
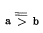
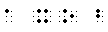
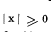
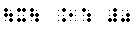
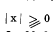
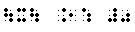
d. Inclusion (is a subset of):
| Bar Over Inclusion |
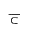 |
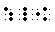 |
| Equals Sign Over Inclusion |
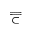 |
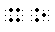 |
| Bar Under Inclusion |
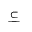 |
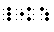 |
| Equals Sign Under Inclusion |
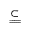 |
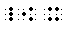 |
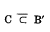
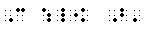
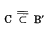
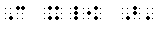
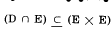
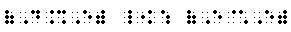
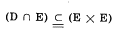
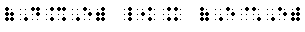
e. Intersection (cap): The intersection sign is a sign of comparison when modified by a bar or equals sign above or below it. An unmodified intersection sign is a sign of operation (see p. xx).
| Bar Under Intersection |
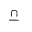 |
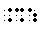 |
| Equals Sign Under Intersection |
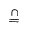 |
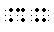 |
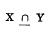
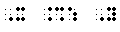
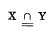
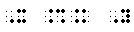
f. Less Than:
Bar Over Less Than
(is equal to or less than) |
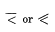 |
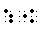 |
Equals Sign Over Less Than
(is equal to or less than) |
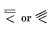 |
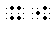 |
Bar Under Less Than
(is less than or equal to) |
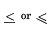 |
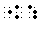 |
Equals Sign Under Less Than
(is less than or equal to) |
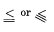 |
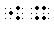 |
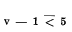
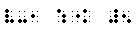
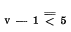
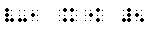
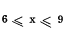
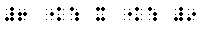
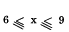
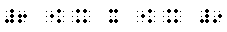
g. Logical Product (meet): The logical product sign is a sign of comparison when modified by a bar or equals sign above or below it. An unmodified logical product is a sign of operation.
| Bar Over Logical Product |
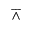 |
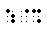 |
| Bar Over and Bar Under Logical Product |
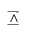 |
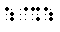 |
| Bar Over and Equals Sign Under Logical Product |
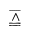 |
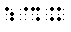 |
| Bar Under Logical Product |
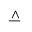 |
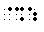 |
| Equals Sign Over Logical Product |
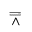 |
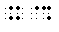 |
| Equals Sign Over and Bar Under Logical Product |
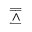 |
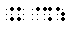 |
| Equals Sign Over and Equals Sign Under Logical Product |
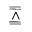 |
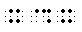 |
| Equals Sign Under Logical Product |
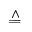 |
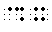 |
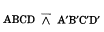
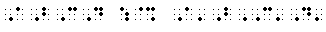
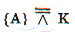
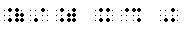
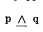
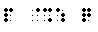
h. Logical Sum (join): The logical sum sign is a sign of comparison when
modified by a bar or equals sign above or below it. An unmodified logical sum is a sign of operation.
| Bar Over Logical Sum |
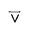 |
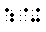 |
| Bar Over and Bar Under Logical Sum |
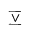 |
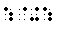 |
| Bar Over and Equals Sign Under Logical Sum |
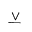 |
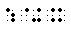 |
| Bar Under Logical Sum |
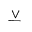 |
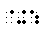 |
| Equals Sign Over Logical Sum |
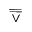 |
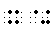 |
| Equals Sign Over and Bar Under Logical Sum |
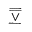 |
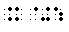 |
| Equals Sign Over and Equals Sign Under Logical Sum |
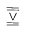 |
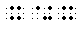 |
| Equals Sign Under Logical Sum |
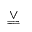 |
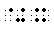 |
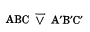
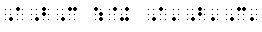
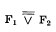
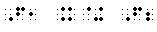
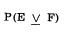
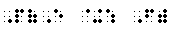
i. Reverse Inclusion:
| Bar Over Reverse Inclusion |
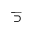 |
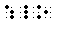 |
| Equals Sign Over Reverse Inclusion |
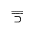 |
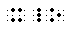 |
| Bar Under Reverse Inclusion |
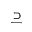 |
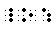 |
| Equals Sign Under Reverse Inclusion |
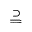 |
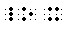 |
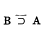
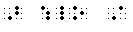
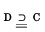
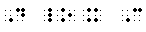
j. Tilde:
| Bar Over Single Tilde |
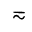 |
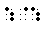 |
| Equals Sign Over Single Tilde |
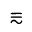 |
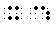 |
Double Tilde
(is approximately equal to) |
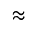 |
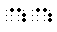 |
| Bar Under Double Tilde |
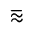 |
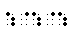 |
| Equals Sign Over Double Tilde |
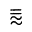 |
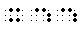 |
| Bar Under Single Tilde |
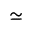 |
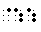 |
Equals Sign Under Single Tilde
(is congruent to) |
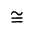 |
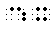 |
| Bar Under Double Tilde |
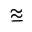 |
 |
| Equals Sign Under Double Tilde |
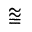 |
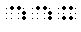 |
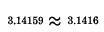
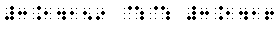
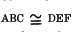
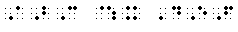
k. Union (cup): The union sign is a sign of comparison when modified by a bar or equals sign above or below it. An unmodified logical sum is a sign of operation.
| Bar Over Union |
 |
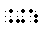 |
| Equals Sign Over Union |
 |
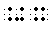 |
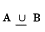
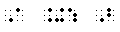
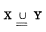
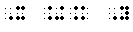
Signs of Comparison Compounded Horizontally: When two or more sign of comparison are arranged side by side, the combination becomes a single comparison sign compounded horizontally. The multipurpose indicator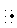 must be placed between the unspaced symbols to indicate that they are printed horizontally, not vertically. Unlisted signs of comparison compounded horizontally must be transcribed according to the same principle. must be placed between the unspaced symbols to indicate that they are printed horizontally, not vertically. Unlisted signs of comparison compounded horizontally must be transcribed according to the same principle.
- Arrows:
Pointing-Up Followed by
Pointing Down |
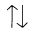 |
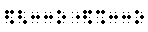 |
Pointing-Down Followed by
Pointing-Up |
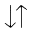 |
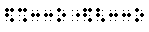 |
Pointing-Up Followed by
Boldface Pointing-Down |
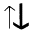 |
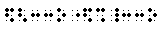 |
Pointing-Down Followed by
Boldface Pointing-Up |
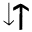 |
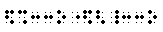 |
Boldface Pointing-Up Followed by
Pointing Down |
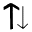 |
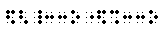 |
Boldface Pointing-Down Followed by
Pointing-Up |
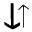 |
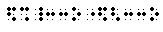 |
Boldface Pointing-Up Followed by
Boldface Pointing-Down |
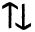 |
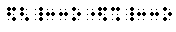 |
Boldface Pointing-Down Followed by
Boldface Pointing-Up |
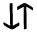 |
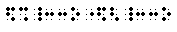 |
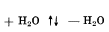
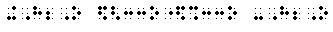
- Greater Than:
| Followed by Less Than |
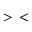 |
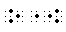 |
Followed by Equals Sign
Followed by Less Than |
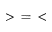 |
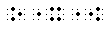 |
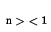
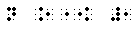
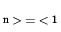
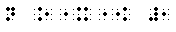
- Less Than:
| Followed by Greater Than |
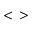 |
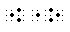 |
Followed by Equals Sign
Followed by Greater Than |
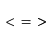 |
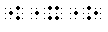 |
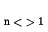
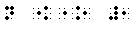
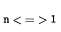
Negated Signs of Comparison: In print, a sign of comparison may be negated by a vertical line of by a slant line drawn through it. In braille, the symbol 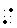 must be used to represent either of the print negation signs and must be placed immediately before the sign of comparison being negated. Negated signs of comparison not illustrated below must be transcribed according to the same principle. must be used to represent either of the print negation signs and must be placed immediately before the sign of comparison being negated. Negated signs of comparison not illustrated below must be transcribed according to the same principle.
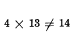
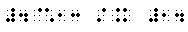
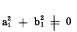
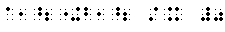
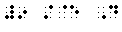
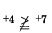
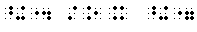
Signs of Comparison and Boldface Type: When it is necessary to show that a sign of comparison is printed in boldface type, dots 4-5-6 are placed before the corresponding symbol in braille. However, the use of dots 4-5-6 to denote boldface type is restricted to the specific signs listed below. They must not be used with any other sign of comparison. Boldface signs of comparison should be used only when the distinction between the regular and boldface forms of the same sign has mathematical significance.
Boldface Arrows
Boldface Equals = 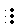
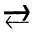 shows that the reaction begins at the left and is completed to the right. shows that the reaction begins at the left and is completed to the right.
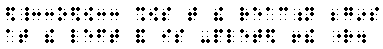
- a X c = b X d if and only if a = c and b = d.
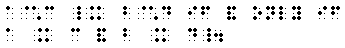
|